Initial Publication Date: March 30, 2007

Advanced Modeling Programs: Introduction to the THERMOCALC Mineral Equilibria Modeling Software

Julie Baldwin, University of Montana; Dexter Perkins, University of North Dakota; and Dave Mogk, Montana State University

What is it?

THERMOCALC is thermodynamic calculation software for tackling mineral equilibria problems. It has two main components: the application itself, and the internally-consistent thermodynamic dataset it uses. The mineral equilibria problems that can be addressed with THERMOCALC include inverse modeling ones (geothermometry/barometry using average PT), and forward modeling ones (calculating phase diagrams for model systems). For the latter there exists a program - drawpd - that allows manually assembled THERMOCALC output to be drawn in postscript.

Principles

In the application of equilibrium thermodynamics to the calculation of phase diagrams, there are two approaches that can be followed: one based on the minimization of Gibbs energy, the other being a derivative equivalent based on the solution of sets of non-linear equations. The main non-linear equations involved are the "equilibrium relationships": the relationships for balanced chemical reactions between the end-members of phases that are in equilibrium with each other:

Transparent 1-pixel GIF0 = ΔGo +RT ln K

In this, ΔGo is the Gibbs energy of the reaction between the pure end-members in the same structure as the phases in which they occur, K is the equilibrium constant, in terms of the activities of the end-members in their phases, T is temperature, and R is the gas constant. THERMOCALC follows this non-linear equation approach.

Applications

Phase Diagram Calculations

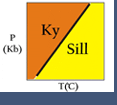
Projections show stable invariant points and univariant reaction lines for all of the bulk compositions in a model system (e.g. petrogenetic grids) (Fig. 1). Compatibility diagrams show the mineral assemblages and ranges of mineral solid solutions at a specified P and T, for all the bulk compositions in the model system (e.g. AFM diagrams) (Fig. 2). Pseudosections show just those phase relationships for a specified bulk composition (Fig. 3). Axes can be P and T, or may reference a particular bulk composition line X (Fig. 4).

This is an image of a P-T projection
Fig. 1. P-T projection for KFMASH (+mu + q + H2O); the in-excess phases are not included in the reactions labeling the univariant lines, as is usual for such diagrams. 
Click image to enlarge.
This is an image of an AFM compatibility diagram
Fig. 2. AFM compatibility diagram for KFMASH (+mu + q + H2O) at P = 6 kbar and T = 560°C. 
Click image to enlarge.
This is an image of a P-T pseudosection
Fig. 3. P-T pseudosection in KFMASH (+mu+q+H2O) for a "common" pelite composition: Al2O3 = 41.89, MgO = 18.19, FeO = 27.29, and K2O = 12.63 (in mol%). 
Click image to enlarge.
This is an image of a T-X pseudosection
Fig. 4. T-x pseudosection in KFMASH (+mu+q+H2O), for a composition line along which FeO:MgO varies, with X = FeO/(FeO+MgO), and Al2O3 = 41.89, FeO + MgO = 45.48, and K2O = 12.63 (in mol%). This composition line goes through the composition used in Fig. 3. 
Click image to enlarge.

Average PT Calculations

With the existence of thermodynamic data for a wide range of end-members in rock-forming minerals, thermobarometry may involve combining many equilibria to find the PT of formation of a rock. In finding a PT of formation, there is an implied displacement of the equilibria to coincide with this PT. These displacements are mainly made by varying the activities of the end-members of the minerals, in proportion to their uncertainties. As a consequence, the equilibria are constrained to move in a more or less highly correlated way because the equilibria involve overlapping subsets of the end-members. These essential correlations should be included in any thermobarometry calculations. Such an optimal approach allows PT, their uncertainties, and a range of diagnostics for outlier identification, to be calculated in a computationally inexpensive way.

Strengths & Limitations

Univariant reactions in a petrogenetic grid are extremely useful in providing bounding constraints on the stability of mineral assemblages. However, petrogenetic grids, especially complex ones with lots of reactions, can be difficult to interpret, and most mineral assemblages in real rocks are higher variance. Moreover, for specific rock compositions, many of these reactions are not 'seen' by a particular bulk composition.

Most people are interested in specific mineral assemblages in the rocks they are studying, for which they have collected and analyzed. The advantage of the pseudosection approach is that this type of diagram portrays only the reactions we are interested in by examining a compositional slice through the full multi-component chemical system.

One caveat of the pseudosection approach is the choice of bulk composition. This can be done via a whole rock geochemical (XRF) analysis if equilibrium is achieved on the 'rock' scale. However, many metamorphic rocks preserve chemical zoning of porphyroblasts and the choice of an 'effective' bulk composition is more appropriate, by combining mineral chemistry data with modal proportions (e.g. by only including garnet cores and excluding the rims).

Worked Examples

Phase Diagrams

The basic approach to setting up a problem in THERMOCALC involves the following sequence of steps:

  1. Choose a model system in which to do the calculations
  2. Formulate the thermodynamics (a-X relationships) of the phases in the system
  3. Decide which phase diagrams are to be constructed and, for pseudosections, choose an appropriate bulk composition:
  4. Build up the phase diagram via calculations on the equilibria involved

Average PT

References

  • Holland, TJB, & Powell, R, 1998. An internally-consistent thermodynamic dataset for phases of petrological interest. Journal of Metamorphic Geology 16, 309-344.
  • Holland, TJB, & Powell, R, 2003. Activity-composition relations for phases in petrological calculations: an asymmetric multicomponent formulation. Contributions to Mineralogy and Petrology 145, 492-501.
  • Powell, R, 1978. Equilibrium Thermodynamics in Petrology Harper and Row, 284 pp.
  • Powell, R, Guiraud, M, & White, RW, 2005. Truth and beauty in metamorphic mineral equilibria: conjugate variables and phase diagrams. Canadian Mineralogist, 43, 21-33.
  • Powell, R, & Holland, TJB, 1988 An internally consistent thermodynamic dataset with uncertainties and correlations: 3: application methods, worked examples and a computer program. Journal of Metamorphic Geology 6, 173-204.
  • Powell, R, & Holland, TJB, 1994. Optimal geothermometry and geobarometry. American Mineralogist 79, 120-133.
  • Powell, R, Holland, TJB, & Worley, B, 1998. Calculating phase diagrams involving solid solutions via non-linear equations, with examples using THERMOCALC Journal of Metamorphic Geology 16, 577-588.
  • Worley, B, & Powell, R, 1999. High-precision relative thermobarometry: theory and a worked example Journal of Metamorphic Geology 18, 91-102.

Related Links

Teaching Activities

  • Multi-equilibrium Thermobarometry Lab (Microsoft Word 53kB Mar29 07) - This Excel-based one week exercise, provided by Dave Pattison at the University of Calgary, includes problems sets involving multi-equilibrium thermobarometry using TWQ and ThermoCalc's 'AvePT' module ('Optimal thermobarometry').

Roger Powell's THERMOCALC Short Course, 2006

These are the files of presentations and problem sets presented at the Granulites 2006 short course in Sao Paulo, Brazil.

Short Course Lecture Presentations

Documents, Tutorials, Examples